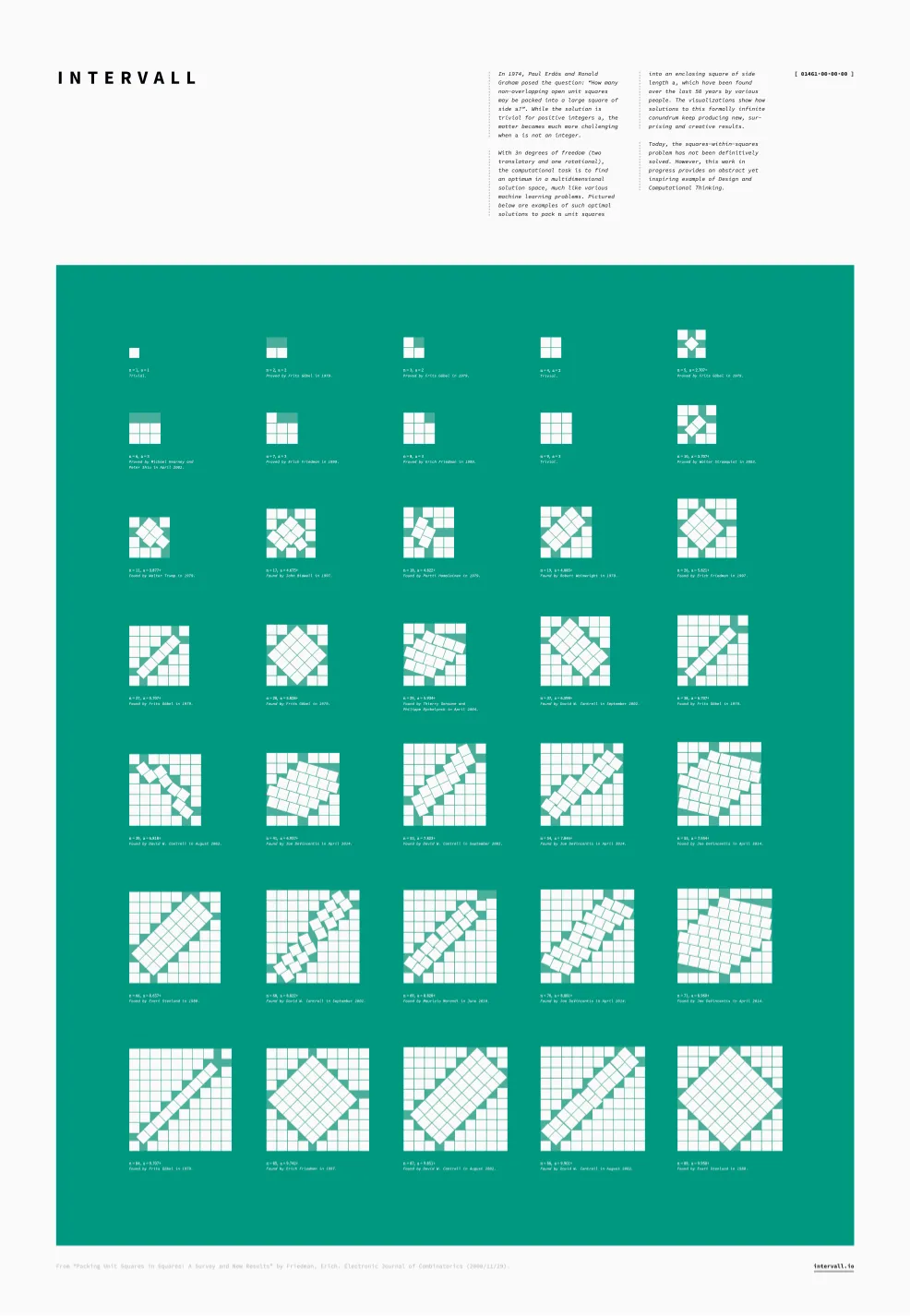
In 1974, Paul Erdös and Ronald Graham posed the question: “How many non-overlapping open unit squares may be packed into a large square of side a?”. While the solution is trivial for positive integers a, the matter becomes much more challenging when a is not an integer.

With 3n degrees of freedom (two translatory and one rotational), the computational task is to find an optimum in a multidimensional solution space, much like various machine learning problems. Pictured below are examples of such optimal solutions to pack n unit squares into an enclosing square of side length a, which have been found over the last 50 years by various people. The visualizations show how solutions to this formally infinite conundrum keep producing new, surprising and creative results.

Today, the squares-within-squares problem has not been definitively solved. However, this work in progress provides an abstract yet inspiring example of Design and Computational Thinking.